Suppose you are trying to cool down a beverage. Will the room-temperature soda you bought be cool in time for your party? Now you can calculate how long it will take the beverage to reach the temperature of the refrigerator. All you need to do is apply Newton’s law of cooling.
Newton’s law of cooling states that the rate at which an object cools is proportional to the difference in temperature between the object and the object’s surroundings. Simply put, a glass of hot water will cool down faster in a cold room than in a hot room. This simple principle is relatively easy to prove, and the experiment has repeatable and reproducible results. This experiment is also a great opportunity for a cross-curricular lesson involving physics and advanced math courses such as Algebra II, Pre-Calculus, and Calculus.
Thermodynamics
Background
Students will need some basic background information in thermodynamics before you perform these activities. Students should be familiar with the first and second laws of thermodynamics.
The first law of thermodynamics is basically the law of conservation of energy. This means that energy can change form. The hot water that you use for this experiment contains heat, or thermal energy. When you used a stove, microwave, or hot plate to heat the water, you converted electrical energy into thermal energy. The total amount of energy in the universe is constant. The energy can change form, but the total amount remains the same. Energy is conserved.
The second law of thermodynamics states that the entropy, or disorder, of the universe always increases. For purposes of this experiment, this means that heat always travels from a hot object to a cold object. If your soup is too hot and you add some ice to cool the soup, the cooling does not happen because “coldness” is moving from the ice to the soup. Rather, the heat from the soup is melting the ice and then escaping into the atmosphere. In this experiment, the heat from the hot water is being transferred into the air surrounding the beaker of hot water.
There are three methods by which heat can be transferred.
- Conduction occurs when there is direct contact. Touch a hot stove and heat is conducted to your hand.
- Convection occurs when there is a bulk movement of fluid (a fluid means a liquid or a gas). Use a fan to cool off, and the heat is carried from you to the surrounding air by convection.
- Radiation is the transmission of heat in the form of waves. Stand in the sunlight, and you will feel the heat transmitted from the sun by radiation.
Newton’s law of cooling applies to convective heat transfer; it does not apply to thermal radiation.
Newton’s law of cooling states that the rate of heat exchange between an object and its surroundings is proportional to the difference in temperature between the object and the surroundings. Mathematically that is represented as:

This can also be expressed as the following equation:

There are 2 general solutions to this equation. If the temperature of the object, T, is greater than the temperature of the surroundings, Ta, then:


Equation 1:

If the ambient temperature, Ta, is less than the temperature of the object, T, the solution to the equation is:


Equation 2:

The solution to the differential equation gives 2 exponential functions that can be used to predict the future temperature of the cooling object at a given time, or the time for an object to cool to a given temperature.
In this experiment, a glass of hot water will cool to match the temperature of the surroundings, and the following equation will be used:
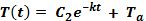
Materials
- Water
- Beaker, 250 mL
- Thermometer
- Hot Plate
- Ice Bath or Refrigerator
- Scientific Calculator
- Graph Paper or Computer with Spreadsheet Software
Safety
Observe all standard lab safety procedures and protocols. Wear appropriate personal protective equipment (PPE). This lab involves using a hot plate and hot water. Wear safety glasses when heating and moving hot water, and use tongs or heat-resistant gloves to move the hot beaker. Turn off and disconnect the hot plate when heating is complete, and remember always to treat the surface of the hot plate as if it were hot.
Procedure
Activity 1: Graph and analyze data for cooling water
- Use a thermometer to measure the temperature of the atmosphere. Record that information as Ta in Table 1. (Note: Alternatively, a probeware system with a temperature sensor can be used to collect data.)
- Heat approximately 200 mL of water in the beaker. Set the beaker on a lab table, insulated from the table surface, where it will not be disturbed.
- Use the thermometer to record the temperature of the hot water. Record that value as T(0) in Table 1.
- Start the timer and continue to record the temperature every 10 minutes. Record this data in Table 1.
Repeat the procedure, measuring the temperature outside, of your ice bath, or in your refrigerator for Ta. Use the same volume of hot water, starting at the same temperature. Record the data in Table 1.
Graph and compare your results. Graph temperature on the y axis and time on the x axis.
If you use a spreadsheet to graph the data and add a trend line, select “exponential function.”
Note: Convert from °F to °C if necessary. °C = (5/9)(°F – 32)
| Temperature (°C) | Temperature (°C) | Time (minutes) |
|---|---|---|
| T(0) | T(0) | 0 |
| T(10) | T(10) | 10 |
| T(20) | T(20) | 20 |
| T(30) | T(30) | 30 |
| T(40) | T(40) | 40 |
| T(50) | T(50) | 50 |
| T(60) | T(60) | 60 |
Questions for Activity 1
- What is the difference in the line representing the water cooling in the classroom and the water cooling in the refrigerator/outside?
- How does the graph tell us if our hypothesis is correct or not?
- What is the independent variable in this experiment?
- What is the dependent variable in this experiment?
- What are some of the controls used in this experiment?
- What other factors could affect the results of this experiment?
Sample Data and Answers
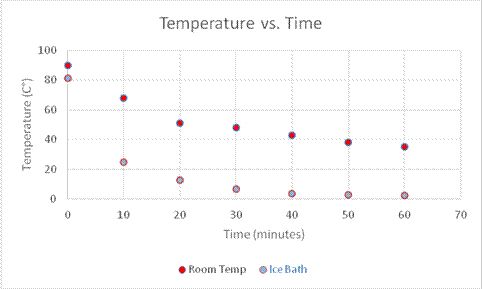
Answers for Activity 1
- As the line on the graph goes from left to right, the temperature should get lower. If Newton’s law of cooling is correct, the line representing the cooler atmosphere should decrease faster.
- The data indicates that the sample of water located in the atmosphere with the cooler temperature cools faster. This agrees with Newton’s law of cooling.
- The independent variable is temperature.
- The dependent variable is time.
- Some controls could be: the substance (water), the mass of the substance (200 mL = 200 g of water), the container, the temperature of the atmosphere, a stable atmosphere (no temperature change or convection currents from a fan or open window).
- Factors that could be changed include: starting at a hotter or colder temperature, using a different mass of water, using a different container (such as a Thermos® or foam cup), or using a different substance (such as a sugar solution or a bowl of soup). You could also try the experiment with a cold liquid and a hot atmosphere, like a glass of cold water warming on a hot day.
Activity 2: Working with the equation for Newton’s law of cooling
This activity is a mathematical exercise. Apply Equation 2 to the data collected in Activity 1 in order to predict the temperature of the water at a given time.
When the temperature of the water or substance that is cooling, T, is greater than the temperature of the surrounding atmosphere Ta¸ the solution to this equation is:
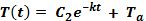
Temperature as a function of time depends on the variables C2, k, and Ta. If these values are known, then the temperature at any time, t, can be found simply by substituting that time for t in the equation. Or the time for an object to reach a certain temperature can be found by solving for t, and substituting T(t) for the given temperature.
Consider the following set of data for a 200-mL sample of water that is cooling over an hour.
| Temperature (°C) | Temperature (°C) | Time (minutes) |
|---|---|---|
| Ta | 25 | |
| T(0) | 72 | 0 |
| T(10) | 59 | 10 |
| T(20) | 52 | 20 |
| T(30) | 46 | 30 |
| T(40) | 40 | 40 |
| T(50) | 37 | 50 |
| T(60) | 29 | 60 |
Starting with the exponential equation, solve for C2 and k.
Find C2 by substituting the time and temperature data for T(0).
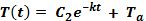
At t = 0, the temperature is 72. When t = 0, e-kt becomes 1.

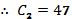
Now use another data point to find the value for k.
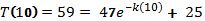
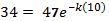
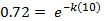
To find the value of k, take the natural log of both sides:
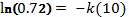
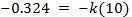
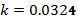
Now use these 2 constants to predict the temperature at some future time, and use the data in Table 1 to verify the answer.
Try to find the temperature at time t = 40 minutes.
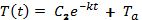
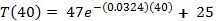
Use a calculator to find the value:
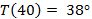
This is close to the sample date in Table 2.
Now try to predict how long it will take for the temperature to reach 30°.
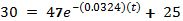
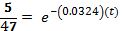
Taking the natural log of both sides:

Solving for t:
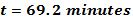
Details for deriving Equations 1 and 2
Students with some experience in calculus may want to know how to derive Equations 1 and 2.
Begin solving the differential equation by rearranging the equation:
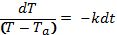
Integrate both sides:

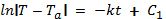
By definition, this means:
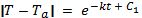
Using the laws of exponents, this equation can be written as:
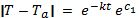
The quantity eC1 is a constant that can be expressed as C2.
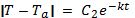
There are 2 general solutions for this equation. One solution is if the matter at temperature T is hotter than the ambient temperature Ta. Since the expression on the left side of the equation is between absolute value bars, (T – Ta) can either be positive or negative.
The solutions, as stated earlier, are given by:
Equation 1:

Equation 2:
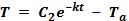
Equation 1 applies if the temperature of the object or substance, T, is greater than the ambient temperature Ta; Equation 2 applies if the ambient temperature is greater than the object or substance.
What if the temperature of the atmosphere is warmer than the sample of matter? How long will a glass of lemonade stay cold on a summer’s day?
In the case that the atmosphere is warmer than your material, the solution for Newton’s law of cooling looks like this:

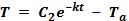
Can you develop a procedure to test this equation? Start with a sample of cold water, and repeat the process in Activity 2. Try to predict how long it will take for the water to reach room temperature.